Blocks, Bricks and Boxes
 Find a cuboid (with edges of integer values) that has a surface area of exactly 100 square units.
Find a cuboid (with edges of integer values) that has a surface area of exactly 100 square units.
Is there more than one?
It is quite easy to find a few solutions. The big challenge is to find all possible solutions.
Can you provide a convincing argument that you have found them all?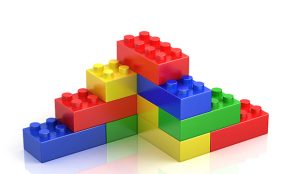
What do you notice about this construction?
What do you notice about the dimensions of these building blocks?
 Find the dimensions of a standard house brick in your country. What do you notice about the width and the length of bricks? Look at the way a wall is built. Why do you think bricks are designed with these dimensions?
Find the dimensions of a standard house brick in your country. What do you notice about the width and the length of bricks? Look at the way a wall is built. Why do you think bricks are designed with these dimensions?
BLOCKS, BRICKS AND BOXES GAME FOR THE WHOLE CLASS
At the start of the game decide whether players will join in the game individually or compete in pairs or small groups against other groups.
The players must keep a record of the dimensions of cuboids whose surface areas have been calculated.
Start with a target surface area, say 100 square units. Players try to find cuboids with edges of integer values that have a surface area of exactly the target area. A fixed time (for example 2 minutes) is given for each round of the game. The winner is the player who gets the most points. The winner chooses the target surface area for the next round.
At the end of each round, for new results not considered already:
- 10 points are awarded for a bulls eye “100”,
- 5 points for each surface area between 95 and 105,
- 2 points for surface areas between 90 and 95 or between 105 and 110.
Competition between groups works well if miscalculated results lose points giving motivation for peer checking and helping each other.
At the end of a game players should think about what they have achieved. Did they find all possible solutions? Which methods and ideas were most useful? What aspects of the problem remain unanswered?
Click here to download the poster.
Click here to download the BRICKS, BLOCKS AND BOXES worksheet.
Click here to download the BRICKS, BLOCKS AND BOXES Inclusion and Home learning Guide.
Click here for Notes for Teachers.
Thank you to https://www.longbeachconcreteservice.com/ for permission to use the image of the bricklaying.
We invented this game when we adapted the Cuboids problem from NRICH with permission of the University of Cambridge.
South Africa COVID-19 News
Here is the official website for COVID-19 updates.
Login
SUPPORT AIMSSEC





