Sierpinski Number and Shape Patterns

There are many investigations and projects you could do based on the Sierpinski Tetrahedron.

For a start: how many small tetrahedra, like the blue model shown, were used to make the 6.5 metre high red balloon model shown below.

Attempt to build the world’s largest balloon Sierpinski tetrahedron at the Grafton Centre. The End result

The smallest tetrahedron  (Stage 0), the blue model,  is made from 6 balloons, each 25 centimetres long.

The green (Stage 1 – 50cm) tetrahedron, made from four 25 cm pyramids, would have edges of length 50 centimetres in a perfect model.

Four of the Stage 1  tetrahedra are used to make the Stage 2 – 1 metre model, and 4 of those to make the next model (Stage 3 – 2 metres) and so on, and so on.  Four tetrahedra are used to make a bigger tetrahedron at each stage of the construction. The lengths of the edges are double the lengths of the edges at the previous stage – so we call this a linear scale factor of 2.

This white 3D printed model shows the construction of the red balloon model.

The balloon model was made from 1024 small tetrahedra with edges of length 25 centimetres. Clearly it was not a perfect shape like the white model but, if it had been perfect, the edges would have measured 8 metres.

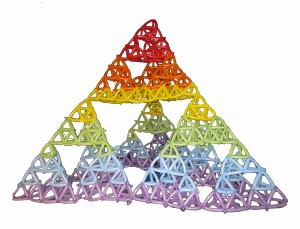
The rainbow tetrahedron shown below  is a Stage 4 – 4 metre construction made with 4 of the Stage 3 – 2 metre tetrahedra.

There are many other questions about number patterns and geometry that you can investigate based on this structure. Let us know what you find out.

Click here to download SIERPINSKI NUMBER & SHAPE PATTERNS worksheet

Click here to download the SIERPINSKI Inclusion and Home Learning Guide.

Click here to download AIMSSEC Teacher Notes SIERPINSKI NUMBER & SHAPE PATTERNS.

These pyramids are on a glass table so you can see their reflections. Describe what you see. You can also play the Youtube video entitled Sierpinski Dream by Mehrdad Garousi.

.

Thanks to Mehrdad Garousi for the video and to Gayla Chandler for the image from her webpage

http://www.fractalnature.com/sierpinskitetrahedron.html