Currently viewing the tag: "Geometric patterns"
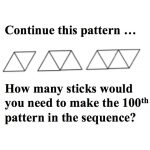
1. If the red square has area 1 square unit. What is the area enclosed by the stage 1 squareflake?
2. What is the area enclosed by the stage 2 squareflake? What about the stages 3 and 4 squareflakes?
3. If replacing each line segment on the edge with the zig-zag is […]
Describe the picture? What do you notice? Talk with your friends about it. What shapes can you see in it?
Describe the different symmetries. Draw the picture yourself. Draw your own designs using this idea. Can you explain how this 2-dimensional picture appears to show a 3-dimensional object?
You could use paperclip […]
Copy this pattern. You could use toothpicks or paper sticks or draw the pattern on isometric paper (download here).
Make or draw the next 3 patterns in the sequence. How would you explain to someone else on the telephone how to do this when you could not point to the diagram?
[…]
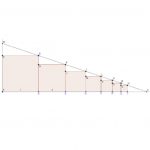
The squares in the diagram are to help us visualise an infinite geometric sum and we are not summing the areas of the squares, simply the lengths along the x-axis. The squares have side lengths given by powers of r: where 0 < r < 1 .
These ‘trisquares’ are made up of three squares and each has an area of 3 square units.
Can you fit them together to make an enlargement of the shape? What is its area?
Can you fit trisquares together to make enlargements of scale factors 3, 4 and 5? What are their areas?
Is it […]
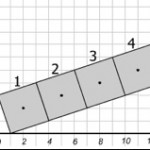
Imagine this pattern of squares continuing on and on …
How do you find the coordinates of the centre of square 1 if you know that (0, 3), (3, 4), (4, 1) and (1, 0) are vertices?
What are the coordinates of the centre of the 20th square?
Imagine the sequence of […]
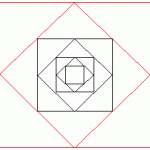
Seven squares are drawn inside each other. The centre points of each side of the outer square are joined to make a smaller square inside it and so on.
The centre square has the area of 1 (one) square unit.
Draw the diagram. You can download square dotty paper here.
What is […]
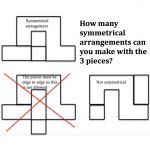
In how many ways can you fit all three pieces together, edge to edge, to make shapes with line symmetry?
The first of these examples shows a symmetrical arrangement, the second is not allowed because the pieces are not placed edge to edge and the third is not symmetrical.
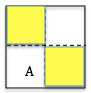
In how many ways can you half cover one big square using two smaller yellow squares as in diagram A.
What are the similarities and differences between the patterns?
Suppose you half cover four big squares by half covering each square as in diagram B.
Diagram B is unchanged when you rotate […]
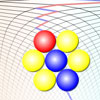
A container holds 4 yellow balls, 2 blue balls and a red ball. The balls are identical in all ways except colour. When you shake the container the balls settle into a hexagonal pattern as shown in the diagram.
You win if two blue balls touch.
How many different ways can […]
FOLLOW US ON SOCIAL MEDIA
