Graph $y={ x }^{ 2 }-2x-1$ .
1. What type of an equation is this?
2. Remember the general form of an equation of this nature: $y={a x }^{ 2 }-bx-c$
When $a > 0$ the graph is $\cup$ shaped and when $a < 0$ it is $\cap$ shaped. What is the situation in our problem?
3. Does the graph of $y={ x }^{ 2 }-2x-1$ intersect with the x-axis? What is the value of y when this graph crosses the x-axis? Can you solve ${ x }^{ 2 }-2x-1=0$ ?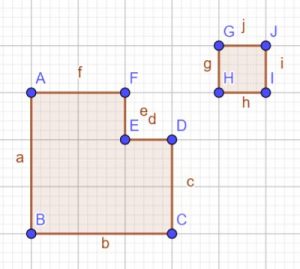
Think of the method of completing a square for solving quadratics. Geometrically, you are thinking of adding shape GHIJ to the bigger shape to make it a complete square, then solve the problem. What are the two values of $x$ which are solutions to ${ x }^{ 2 }-2x-1=0$?
4. From ${ x }^{ 2 }-2x-1 = {(x – 1)}^{2} – 2 = 0$, notice that the graph is above the x-axis for ${(x – 1)}^{2}\ge 0$ for all values of $x$. $\therefore$ The minimum value of ${(x – 1)}^{2}$ is zero and this happens when $x – 1 = 0$ $\rightarrow$ $x = 1$. What is the value of y when $x = 1$? Comment on this point.
5. Parabolas are symmetrical shapes, what is the line of symmetry of this graph?
6. At what point(s) does the graph cross the $x$- axis?
7. Taking into consideration (accommodating) all the special points you have obtained so far, draw a Cartesian plane. Plot all the special points. Graph $y={ x }^{ 2 }-2x-1$.
Click here to download the GRAPHING QUADRATICS poster.
Click here to download the GRAPHING QUADRATICS worksheet.
Click here for Notes for Teachers.
If you enjoyed this article, please consider sharing it!
FOLLOW US ON SOCIAL MEDIA
