Posts by: toni
Investigations of angle properties of parallel lines, similarity in 2 and 3 dimensions and scale factors for linear, area and volume enlargement.
Click here to download a pdf with all you need to run your own professional development workshop.
These counting games lead to an understanding of multiples and common multiples, number patterns and the Sieve of Eratosthenes to find prime numbers.
Click here to download a pdf with all you need to run your own professional development workshop.
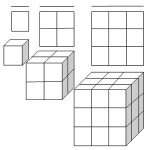
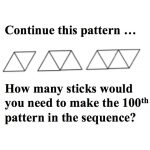
Copy this pattern. You could use toothpicks or paper sticks or draw the pattern on isometric paper (download here).
Make or draw the next 3 patterns in the sequence. How would you explain to someone else on the telephone how to do this when you could not point to the diagram?
[…]
JUST A MINUTE
How long is a minute? What can we do in a minute?
You will need: a stopwatch, paper & pencils (or other recording implements).
Activity 1: Estimate a minute
Sit with your head on your arms so that you will not be distracted. When the timekeeper says […]
If you are at row 0 column 1 (0 ; 1) how many columns do you go across and how many rows back would you go to get to row 2 column 4 (2 ; 4)?
Give directions for getting from row 1 column 0 (1 ; 0) to row 2 column […]
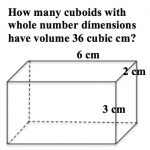
What is the volume of this cuboid?
How many other cuboids with whole number dimensions have the same volume?
Do any of them have the same surface area?
Which has the biggest surface area? Which has the smallest?
How many have a square cross section?
Click here for Notes for Teachers.
[…]
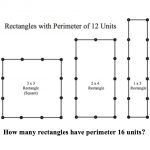
This activity is about rectangles with whole number dimensions. With other lengths and breadths there are infinitely many possible rectangles so here we just stick to whole numbers.
How many rectangles can you make with perimeter 12 units?
What do you know about rectangles? What is the smallest rectangle that you can […]
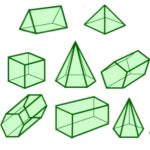
A tetrahedron and an octahedron, both have equilateral triangular faces.
Can you arrange these 8 polyhedra in a line so that every two polys next to each other have a face of the same shape. The matching faces do not need to be the same size.
What do you notice about this diagram.
Draw the diagram accurately starting with a regular pentagon.
Find all the angles in the diagram.
Label 4 sets of congruent triangles. Which triangles are similar?
Describe the symmetries of the shape (that is the transformations that map the shape onto itself).
Describe the symmetries […]
Choose pairs of these numbers to make the total of each pair as close to eleven thousand as possible?
Which total is closest to eleven thousand?
Which pair of numbers has the largest total?
What number would you add to each of these 8 numbers to make eleven thousand?
You might […]
FOLLOW US ON SOCIAL MEDIA
