Posts by: toni
In front of you there are 20 envelopes.
Eight of the envelopes each contain 5 blue and 3 red sheets of paper.
The other 12 envelopes each contain 6 blue and 2 red sheets of paper.
You choose one envelope at random. Then you choose a sheet of paper from it at […]
This stand is made from cylindrical wooden dowel of diameter 1 cm joined at the corners with 45 degree mitres.
The external dimensions of the stand are 10 cm by 10 cm.
What are the volume and surface area of the stand?
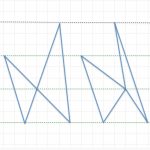
The sketch below shows how I marked […]
Find the total area of the two red triangles by two different methods if ACD and BCE are straight lines .
What are the lengths PC and CQ?
The triangles are re-drawn with PC = x cm and CQ = y cm keeping the total area the same and keeping the distances between the green […]
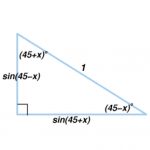
Draw a triangle with angles x, (45 + x) and (45 – x) degrees.
What does Pythagoras Theorem tell you about these angles?
Use this information to find this sum of squares of sines:
Click here to download the DEGREE CEREMONY worksheet.
See the AIMSSEC Notes for Teachers.
[…]
Put the missing symbol into the box to make these number sentences correct.
Use the symbols +, −, ×, ÷ and =.
17 ☐ 21 = 38
17 ☐ 3 = 51
57 ☐ 29 = 28
9 ☐ 63 ÷ 7
You can make most of the number sentences below correct by putting in […]
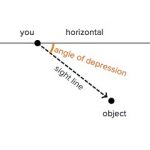
Two ships are heading towards a lighthouse on the same path, one behind the other. From a height of 42 metre the closer ship is observed at an angle of depression θ where tan θ = ⅘ and the other ship at an angle of depression of 30 degrees. Draw a diagram.
[…]
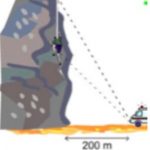
A climber is stuck on a cliff face. A rescue worker on the ground is 200 m from the bottom of the cliff. The angles of elevation of the climber and of the top of the cliff as seen by the rescuer are 45 degrees and 60 degrees respectively. Draw a diagram.
[…]
Put these temperatures in order from the coldest to the hottest:
– Boiling water – Normal temperature of human body – Ice – The sun – Water in a swimming pool – Temperature in the shade on a hot summer day.
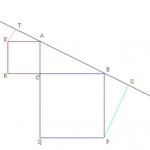
In the diagram ASRC and BCQP are squares and PU and ST are perpendicular to the straight line TABU.
Prove that PU + ST = AB.
Here are three views of the same cube.
One face (not shown) is plain yellow.
The other five faces have squares on them coloured blue, orange, pink, green and red.
Which face is opposite the blue one?
Draw a net of this cube and label the six faces.
FOLLOW US ON SOCIAL MEDIA
