Currently viewing the tag: "Triangles"
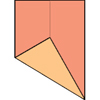
This is a classic Chinese Tangram. Each of the people illustrated, and thirteen convex polygons, can be made with the 7 pieces and there are hundreds of other puzzles based on this tangram.
Can you find a way to fold a sheet of paper so that you can cut out the tangram pieces […]
Arrange the 7 tangram pieces to make the shape in the grey picture.
What do you notice about the pattern? Draw the pattern and make a list of what you see in it.
Describe the shapes of the tangram pieces.
What is the same and what is different about the shapes of […]
Why does this fold create an angle of sixty degrees?
Make a centre crease down the length of the paper then open it up.
Next fold one corner over and onto the centre crease so that the fold line passes through the corner next to it (on the short side of the paper).
You […]
Take any triangle and label it ABC with A as the largest angle. Fold the triangle along PQ, PS and QT where the points P and Q are the midpoints of AB and AC and PS and PT are perpendicular to BC.
What do you notice? Why does this happen? Will it […]
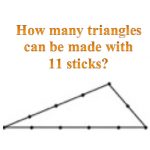
Use 11 sticks of equal length to make this triangle with edge lengths 2, 4 and 5. You might like to record this as (2, 4, 5). How many other triangles can you make with 11 sticks?
You could use paper sticks, toothpicks, or a piece of string with […]
There are many investigations and projects you could do based on the Sierpinski Tetrahedron.
For a start: how many small tetrahedra, like the blue model shown, were used to make the 6.5 metre high red balloon model shown below.
The smallest tetrahedron (Stage 0), the blue model, is made from 6 balloons, each […]
See the Bendy Quads video https://bit.ly/BendyQuadsVideo
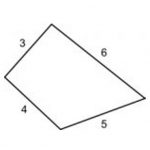
Four rods are hinged at their ends to form a convex quadrilateral with edges of length 3, 4, 5 and 6 (in that order). Investigate the different shapes that the quadrilateral can take if the polygon is always convex.
How do the angles change […]
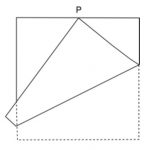
A square of paper 8 cm by 8 cm is folded so that the corner P coincides with the midpoint of an opposite edge as shown in the diagram.
Investigate the three triangles (where there is a single thickness of the paper) that are formed by folding in this way.
Click here for […]
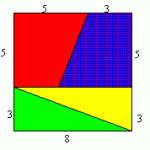
Look at this square divided into four pieces : two identical triangles and two identical trapezia.
The edge of length 3 from each triangle matches with the edge of length 3 from a trapezium so that the four pieces from the square now occupy a rectangular space.
The square had side length 8 […]
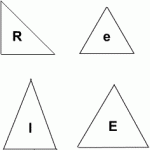
A tetrahedron (plural tetrahedra) is a solid with four triangular faces. How many different tetrahedra can you make using the four different types of triangle shown in the diagram if you have an unlimited number of each type?
Type R are right angled isosceles triangles with sides a, a and b units.
Type […]
FOLLOW US ON SOCIAL MEDIA
