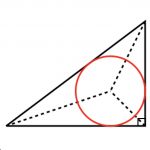
What can you discover by accurately constructing some right angled triangles with integer edge lengths and their inscribed circles (incircles)?
If you make some conjectures, can you prove them?
Share ideas below about what you have discovered.
More ideas about incircles will be posted here. Watch this space.
A fence is built around a square field.
Suppose another fence is built exactly one metre longer so the path between the two fences is the same width all the way round including at the corners of the field.
How wide would this path be?
Would a mouse be able to run along it? […]
A wire belt is tied tightly around the Earth at the equator. Suppose the belt is made exactly one metre longer and held around the Earth at the equator so that it is the same distance away from the Earth everywhere. Would a mouse be able to crawl under the new belt? How do […]
A disc (circle) rolls around the outside edge of a square so that its circumference always touches the edge of the square.
Describe the path (or locus) of the centre of the disc and its length.
Describe the paths of the centres of a disc rolling around the edges of other polygons.
Start to explore ideas of Fibonacci numbers and the golden ratio by copying the picture and drawing this spiral which is made up of quarter circles. Watch this inspirational video. To download the poster click here.
Then create your own elephant. The numbers in the centres of the squares […]
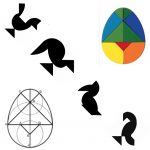
Follow the instructions below to make your own Magic Egg tangram or download the template and cut out the pieces.
You can make many birds from the 9 puzzle pieces of the Magic Tangram Egg.
Break the egg and re-assemble the 9 pieces to hatch one of many varieties of birds.
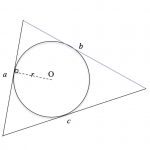
A circle of radius r and centre O, is inscribed in a triangle with edges of lengths a, b and c.
Find the area and circumference of the circle.
Find the area and the perimeter of the triangle.
What do you notice about the ratios of the two areas and the ratios […]
a. Draw 2 circles with equal radii, intersecting at C, so that each goes through the centre of the other.
b. Draw a third circle of the same radius with centre C.
c. Draw 4 more circles with centres on the circle centre A and passing through A.
This baobab tree in Senegal is reported to be 6000 years old.
Try to estimate the distance around the tree trunk at its base measured in metres.
Does this picture give you an idea how you might use the picture, with your class at school, to work out a better estimate […]
The picture shows you how to use a paper clip to draw circles. You just need to open out the paper clip as shown,
Draw a circle and mark 24 points equally spaced around the circumference. To do this, draw a circle and mark the centre, and mark your first point on […]
South Africa COVID-19 News
Here is the official website for COVID-19 updates.
Login
SUPPORT AIMSSEC