Dotty Relations

On the grid join the t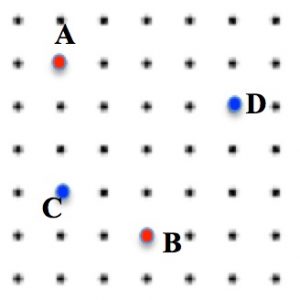wo red dots, A and B, together with a straight line.

Now join the blue dots, C and D, with a straight line.

At what angle do the two lines cross?

Investigate the number of squares “along” and “down” from A to B compared with the number of squares “along” and “up” from C to D. What do you notice?

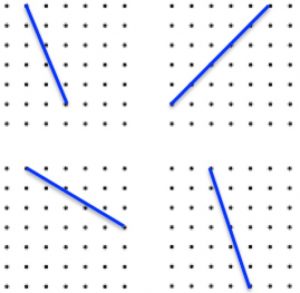Using what you have discovered draw lines on these grids parallel to the lines shown.

Can you find more than one solution each time? Why or why not?

Does it matter how long the lines are? Why or why not?

Now find lines perpendicular (at right angles) to the lines shown. Again can you find more than one solution? Does it matter how long the lines are? Explain.

You may download dotty squared paper for this activity.

See the Notes for Teachers

Click here to download the poster

This problem is adapted from the NRICH task Dotty Relations with permission of the University of Cambridge. All rights reserved.