30-Minute Global Maths and Science Lesson – Beltway Around The Earth – Outer Rims
Click here to register for the 2023 GLOBAL MATHS AND SCIENCE LESSON ON TUESDAY 10 OCTOBER. See below for links to lesson resources.
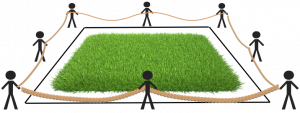
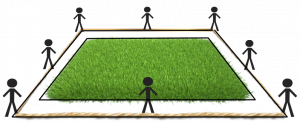
1. Working with a square drawn on the ground in the classroom, or in a bigger space, indoors or outdoors, find the perimeter of the square. The square can be any size. Use string to mark out the line of a fence (string-fence) around the square that is exactly one metre longer than the perimeter of the square. The string needs to be a little more than one metre longer than the perimeter of your square to allow for tying a knot to make the string into a loop that is exactly the right length.
2. Mark the string into 4 equal lengths to give the corners of the square ‘string-fence’ and then hold the 4 corners, stretching the string to make a square. Lower the string close to the ground, adjust the position of the ‘string-fence’ so that the width of the gap between the square and the string is the same all the way round. Then measure the width of the gap.
3. Talk about what would happen to the gap if the experiment is repeated for a smaller square, and again with the string one metre longer than the perimeter of the square. Vote as to whether the gap between the square and the string will be less, the same, or more. You can think of this as making the path between the square and the fence narrower, the same, or wider. Which will it be? Carry out the experiment with the smaller square to find the answer.
4. If you repeated the experiment for a very large square field making an outer ‘string-fence’ again exactly one metre longer than the perimeter of the field, would path between the field and the fence be wide enough for a cow to walk along it? 
 Would a mouse be able to run along the path?
Would a mouse be able to run along the path?
Follow-up 1 NOT-SO-SQUARE FENCE
A fence is built around a square field. Suppose another fence is built exactly one metre longer, so the path between the two fences is the same width all the way round including at the corners of the field.
How wide would this path be?
Would a mouse be able to run along it?
Could a farmer drive his cows along the path between the two fences?
 Follow-up 2 BELT AROUND THE EARTH
Follow-up 2 BELT AROUND THE EARTH
A wire belt is tied tightly around the Earth at the Equator. (Let’s assume the Earth is perfectly spherical and we have no worries about oceans and mountains interfering with our ability to conduct this task!)
Now suppose the belt is made exactly one metre longer and held around the Earth at the Equator so that it is the same distance away from the Earth everywhere. Would a mouse be able to crawl under the new belt? How do you know?
Would a cat be able to crawl under?
Would the answer be the same for the moon?
What assumptions do you need to make to answer this question?
Click here for the 30-Minute Lesson (the Notes For Teachers).
South Africa COVID-19 News
Here is the official website for COVID-19 updates.
Login
SUPPORT AIMSSEC