Posts by: toni
My car has number plate – S208VBJ.
Using my special code S208VBJ adds to 65.
Using the code every character, a digit or a letter, is separate.
Numbers are simply added together so that, in the first number plate 208 is 2 + 0 + 8 = 10.
Letters are translated into […]
If you change the order of these digits you can get 24 different numbers, for example 2547, 2574, 2745, … Can you find all 24 different numbers made by using each of the digits 2, 4, 5, 7 just once?
How many of these numbers have 2 in the thousands place? How many have 2 […]
Using the numbers 1, 2, 3, 4 and 5 once and only once and the symbols × and ÷ once and only once, what is the smallest whole number you can make?
Now suppose you have to use the symbol + once and the symbol ÷ twice what is the smallest fraction […]
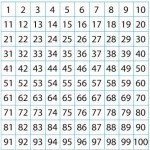
A hundred square has been printed on both sides of a piece of paper.
One square is directly behind the other.
What is on the back of 100? 58? 23? 19?
Can you see a pattern?
Click here for the HUNDRED SQUARE Notes for Teachers.
Click here for the HUNDRED […]
What do the digits in the number 15 add up to?
How many other numbers up to a thousand have digits with the same total if we only include numbers without zeros?
Click here for Solutions and Suggested Lesson Plans in the AIMSSEC Notes for Teachers.
This problem is adapted from the NRICH […]
Little Man is much smaller than you and me. Here is a picture of him standing next to an ordinary mug.
Can you estimate how tall he is? How tall do you think Little Man’s mug might be?
My mug is 10 centimetres tall and it holds 300 millilitres but mugs come in different […]
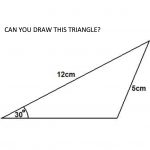
Imagine you have a pencil, ruler, protractor and compass and you try to draw this triangle. Is it possible?
If you have geometry instruments then try it for yourself. What do you notice?
Can you give reasons for your answer to the question “Is it possible?”
Click here for The Notes for Teachers. […]
(STEP 1) Choose any two numbers from the 7 times table. Add them together. What do you notice? Repeat with some other examples, always choosing pairs of numbers from the same times table. What do you notice? Does the same thing always happen? Why or why not?
[…]
Here you see the front and back views of a dodecahedron which is a solid with pentagonal faces.
Using twenty of the numbers from 1 to 25, each vertex can be numbered so that the numbers around each pentagonal face add up to 65.
The number F is the number of faces […]
You are only given the three midpoints of the sides of a triangle.
How can you construct the original triangle?
Is there more than one way to find the answer?
What similarities and differences can you see between this Triangle Midpoints problem, The Checkit Game with Addition https://aiminghigh.aimssec.ac.za/checkit-game/ and Polycircles https://aiminghigh.aimssec.ac.za/polycircles/
FOLLOW US ON SOCIAL MEDIA
